Although much of what has been discussed applies equally to both very long and very short lengths of cable, there are a number of useful simplifying approximations we can make when dealing with the most usual cases where cable lengths are short. In electronic terms we have to define ‘short’ and ‘long’ here relative to the wavelength (along the cable) of the highest frequency in use in the signals of interest. To some extent this is a matter of judgement but a useful rule of thumb is to check if the cable length is at least a couple of orders of magnitude less than a quarter-wavelength. If it is, we can often treat the cable as ‘short’. If not, it may be best to regard it as ‘long’ and employ the full metal jacket of transmission line theory.
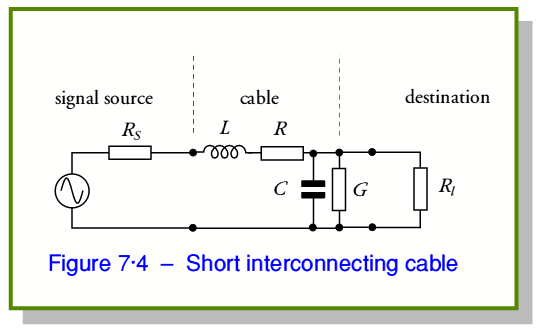
As an example, consider a 1 metre long co-axial cable for use as part of an audio system. Is this cable ‘short’? Well, depending on how good your ears are, you can probably hear sinewaves up to a frequency between 15 kHz and 25 kHz. If we take the upper frequency and assume a velocity along the cable similar to that of EM waves in free space we obtain a length for a quarter-wavelength at 25 kHz of approx 3 km. Hence a 1 m ‘audio interconnect’ is more than three orders of magnitude shorter, so we can treat it as being ‘short’ for most purposes. This means that instead of regarding it as a series of incremental lengths which pass the signal along we can treat it as one ‘lumped’ section placed between the signal source and destination as shown in figure 7·4.
Where for a cable of length,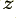 
, we can simply say that
and analyse the effect of the cable as if it were a form of ‘filter’ placed between the signal source and destination. To do this we can use simple a.c. circuit theory although we may need to take into account the fact that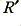 
and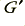 
may be frequency dependent to some extent.
Summary
You should now understand how a cable can be modelled and analysed as a series of incremental lengths in the form of a Transmission Line. That this line may have losses due to physical imperfections of both the conducting and dielectric elements. You should know how the cable’s Characteristic Impedance and the velocity of signal transfer depend upon the cable’s electrical properties. That a cable may be Dispersive, and that dispersion may, in principle, be prevented by arranging for the cable to satisfy Heaveyside’s Condition. You should also understand how the Skin Effect can cause problems with wideband signals, but that this may be combatted using either woven/braided wiring and/or a change of conductor. Finally, you should now be able to see when a cable can be treated as a ‘short’ section and its effect assessed without the need for the full details of transmission line theory.
